Wavelength Calculator
Calculator Use
The wavelength calculator solves for wavelength, velocity or frequency given 2 known variables. Choose a calculation to use the wavelength equation λ = v/f to solve for wavelength λ, velocity v or frequency f.
Enter numbers, decimals or scientific notation as in 4.56e8.
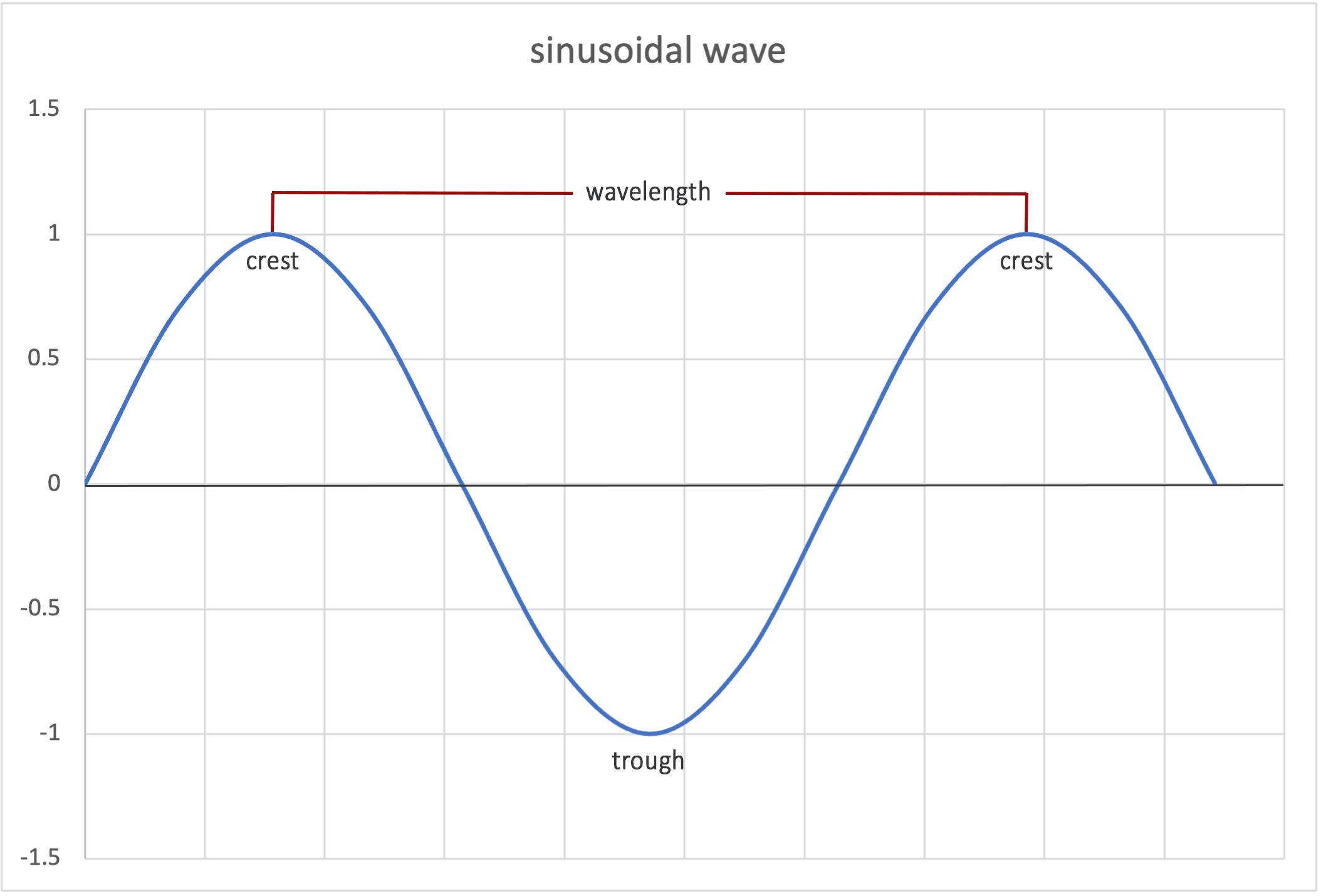
Waves are signals that travel through air, water, light, and even wire as in the case of an electrical AC current. Wavelength is the distance between 2 identical points on a sinusoidal wave. If you know the frequency of a wave traveling through a medium you can calculate how far it is between each crest of the wave or each trough of the wave.
The Wavelength Equation
Variables in the Wavelength Equation
- \( \lambda \) = wavelength
- \( v \) = velocity
- \( f \) = frequency
Wavelength is calculated as the ratio of velocity to frequency, or wave velocity divided by wave frequency. The wavelength calculator uses 2 known values to calculate the third.
Note that the standard units for wavelength are meters, seconds and hertz. If you enter other units of measure the calculator will convert among units for you.
A Note on Significant Figures
For a value of 83456, selecting 4 significant figures will result in 83460. For a value of 0.083456, selecting 4 significant figures will result in 0.08346. For more information see our page on Significant Figures.
Wavelength Formulas
Solvinge for wavelength, velocity or frequency using the following formulas:
Calculate λ Given v and f
Calculate wavelength given velocity and frequency.
Calculate v Given λ and f
Calculate velocity given wavelength and frequency. Find the speed of the wave.
Calculate f Given v and λ
Calculate frequency given velocity and wavelength.
Example: What is the Wavelength of Bell Sounding from a Clocktower?
Suppose there is a clocktower in your town that rings 12 times each day at noon. The town is proud of this clocktower because it has been ringing for a century and they've remarked that the antique bell rings at a frequency of 130 Hz. What is the wavelength of the bell tone?
First assume that the temperature of the air is about 68°F (20°C). Referring to the Velocity Measurements table above you can see that the speed of sound in air is 343 m/s. Given that wavelength is velocity divided by frequency you can now set up and solve the frequency equation.
So the wavelength of the bell tone traveling through air is 2.64 meters.