Sphere Calculator

Basic Calculator

Calculators

Geometry

Solid

Sphere Calculator

Choose a Calculation

Sphere Image

radius r =

Let pi π =

Units

Significant Figures

Answer:

radius

r =

volume

V =

surface area

A =

circumference

C =

In Terms of Pi π

volume

V =

surface area

A =

circumference

C =

Get a Widget for this Calculator

Sphere Shape

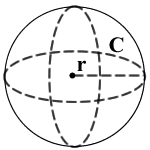
Sphere Diagram with r = radius and c - circumference

r = radius
V = volume
A = surface area
C = circumference
π = pi = 3.1415926535898
√ = square root

Calculator Use

This online calculator will calculate the 3 unknown values of a sphere given any 1 known variable including radius r, surface area A, volume V and circumference C. It will also give the answers for volume, surface area and circumference in terms of PI π. A sphere is a set of points in three dimensional space that are located at an equal distance r (the radius) from a given point (the center point).

Units: Note that units are shown for convenience but do not affect the calculations. The units are in place to give an indication of the order of the results such as ft, ft² or ft³. For example, if you are starting with mm and you know r in mm, your calculations will result with A in mm², V in mm³ and C in mm.

Sphere Formulas in terms of radius r:

Volume of a sphere:
- V = (4/3)πr³
Circumference of a sphere:
- C = 2πr
Surface area of a sphere:
- A = 4πr²

Volume of a Sphere

in terms of
radius

\[ V = \frac{4}{3}\pi r^3 \]

\[ V \approx 4.1888r^3 \]

in terms of
surface area

\[ V = \frac{A^{3/2}}{6\sqrt{\pi}} \]

\[ V \approx 0.09403A^{3/2} \]

in terms of
circumference

\[ V = \frac{C^3}{6\pi^2} \]

\[ V \approx 0.01689C^3 \]

Surface Area of a Sphere

in terms of
radius

\[ A = 4 \pi r^2 \]

\[ A \approx 12.5664r^2 \]

in terms of
volume

\[ A = \pi^{1/3} (6V)^{2/3} \]

\[ A \approx 4.83598V^{2/3} \]

in terms of
circumference

\[ A = \frac{C^2}{\pi} \]

\[ A \approx 0.3183C^2 \]

Radius of a Sphere

in terms of
volume

\[ r = \left(\frac{3V}{4 \pi}\right)^{1/3} \]

\[ r \approx 0.62035V^{1/3} \]

in terms of
surface area

\[ r = \sqrt{\frac{A}{4 \pi}} \]

\[ r \approx 0.2821 \sqrt{A} \]

in terms of
circumference

\[ r = \frac{C}{2 \pi} \]

\[ r \approx 0.1592C \]

Circumference of a Sphere

in terms of
radius

\[ C = 2 \pi r \]

\[ C \approx 6.2832r \]

in terms of
volume

\[ C = \pi^{2/3} (6V)^{1/3} \]

\[ C \approx 3.89778V^{1/3} \]

in terms of
surface area

\[ C = \sqrt{\pi A} \]

\[ C \approx 1.77245\sqrt{A} \]

Sphere Calculations:

Use the following additional formulas along with the formulas above.

Given the radius of a sphere calculate the volume, surface area and circumference
Given r find V, A, C
- use the formulas above
Given the volume of a sphere calculate the radius, surface area and circumference
Given V find r, A, C
- r = cube root(3V / 4π)
Given the surface area of a sphere calculate the radius, volume and circumference
Given A find r, V, C
- r = √(A / 4π)
Given the circumference of a sphere calculate the radius, volume and surface area
Given C find r, V, A
- r = C / 2π